
views
X
Research source
The time value of money distinguishes between present value, the current-day worth of a future value, and future value, the value a certain of money today will have at a specified date in the future. With these two tools, you can calculate a number of other financial concepts.
Calculating Future Value

Know what future value measures. Future value is the value of an asset or amount of money at a specified date in the future. Future value is calculated by multiplying the present value of the asset or amount of money by the effects of compound interest over a number of years. This calculation relies on an interest rate that will be earned by the money or asset over those years.

Learn the future value equation. The future value equation involves only three variables: the principal amount (also called present value), the interest rate, and the number of periods over which the interest will be accumulating. It measures the future value that will be attained through the growth of the principal. The exact equation is as follows: F V = P V ( 1 + r ) n {\displaystyle FV=PV(1+r)^{n}} FV=PV(1+r)^{{n}}. In the equation, the variables represent the following figures: FV is the future value. PV is the present value (the principal). r is the interest rate for each period. n is the number of periods. In many instances, n is a number of years. This is the case when the r used is an annual interest rate.
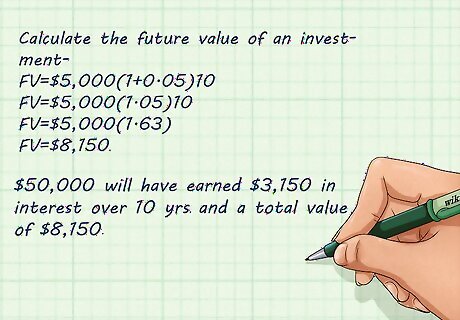
Calculate the future value of an investment. Imagine that you have invested $5000 in an account that earns five percent annual interest. You want to know how much the account will be worth in ten years. Start by inputting all of your variables into the future value equation. Your equation in this example would look like this: F V = $ 5 , 000 ( 1 + 0.05 ) 10 {\displaystyle FV=\$5,000(1+0.05)^{10}} FV=\$5,000(1+0.05)^{{10}} Note that the interest rate, 5 percent, was converted to a decimal in the equation. This was done by dividing by 100 (5/100=0.05). Start the calculation solving the addition in parentheses. Your equation should now look like this: F V = $ 5 , 000 ( 1.05 ) 10 {\displaystyle FV=\$5,000(1.05)^{10}} FV=\$5,000(1.05)^{{10}} Solve the exponent. This is done on a calculator by typing the lower number (1.05 in this case), pressing the exponent button (usually x y {\displaystyle x^{y}} x^{y}), and then entering the higher number (10 here) and pressing enter. Your equation should now look like this: F V = $ 5 , 000 ( 1.63 ) {\displaystyle FV=\$5,000(1.63)} FV=\$5,000(1.63) Note that the result of the exponent, 1.63, is a rounded figure (the actual result is 1.62889...). If you don't round this number off, your later calculations will vary from the example. Solve the multiplication. This gives you F V = $ 8 , 150 {\displaystyle FV=\$8,150} FV=\$8,150 The future value of your $5,000 is $8,150. In other words, your $5,000 will have earned $3,150 in interest over the ten years and will then have a total value of $8,150.
Calculating Present Value

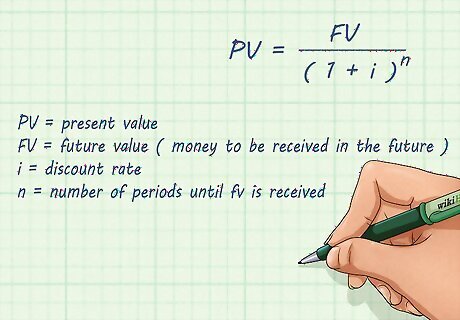
Learn the basics of present value. Present value can be defined as "the current-day value of a future sum of money or stream of cash flows given a specified rate of return (interest rate)." This rate of return, called the discount rate, is used to decrease the future value of the payment or cash to find its present value. Finding the appropriate discount rate is important for properly valuing future cash flows. In simpler terms, present value expresses the reality that a $10,000 payment now is worth more to an investor than a $10,000 payment in five years. Put another way, to find the present value of the future $10,000, we need to find out how much we would have to invest today in order to receive that $10,000 in the future.
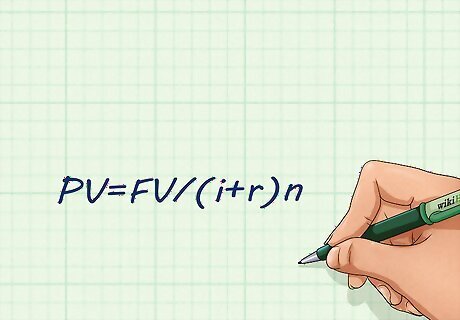
Use the present value equation. The present value is equation is very similar to the future value equation, except the exponent for the number of years is negative. The equation is usually stated as P V = F V ( 1 + r ) n {\displaystyle PV={\frac {FV}{(1+r)^{n}}}} PV={\frac {FV}{(1+r)^{{n}}}}. The variables stand for the following: PV is the present value. FV is the future value. This represents the stated value of the future payment. r is the discount rate. This can be many different relevant rates, especially in corporate finance, but here we are using the interest earned on a compound interest-earning account. n is the number of periods (years in this case).
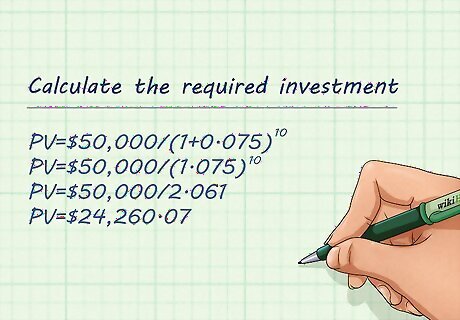
Calculate the required investment to reach a future amount. One of the uses of present value is to determine how much money would need to be put into an account now so that the value of the account reached a certain amount in a number of years. For example, imagine that you are saving for college and wish to have an account value of $50,000 in ten years. The account earns 7.5 percent interest each year. To find the investment needed now to reach this value, input your variables in the present value equation. Your future value is $50,000, n is 10, and r is 0.075 (7.5% expressed as a decimal by dividing by 100). So, your completed equation is: P V = $ 50 , 000 ( 1 + 0.075 ) 10 {\displaystyle PV={\frac {\$50,000}{(1+0.075)^{10}}}} PV={\frac {\$50,000}{(1+0.075)^{{10}}}} Start by adding the 1 to i in the parentheses to get: P V = $ 50 , 000 ( 1.075 ) 10 {\displaystyle PV={\frac {\$50,000}{(1.075)^{10}}}} PV={\frac {\$50,000}{(1.075)^{{10}}}} Next, solve the exponent above the parentheses to get: P V = $ 50 , 000 2.061 {\displaystyle PV={\frac {\$50,000}{2.061}}} PV={\frac {\$50,000}{2.061}} The exponent can be solved on a calculator by inputting the variable in parentheses first, pressing the exponent button (usually x y {\displaystyle x^{y}} x^{y}), and then inputting the exponent and pressing enter. Note that the result, 2.061, is a rounded number. If you do not round this number, you will get a different final result than in the example. Finally, solve the remaining division to get P V = $ 24 , 260.07 {\displaystyle PV=\$24,260.07} PV=\$24,260.07 You only need to invest $24,260.07 in the account now to have $50,000 in ten years.
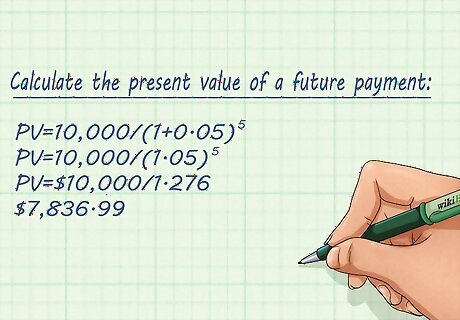
Calculate the present value of a future payment. Imagine that you are going to receive a payment of $10,000 in five years and you want to know how much less this will be worth than if you got the money now. For the discount rate, imagine that you have an account you could put the $10,000 into that would earn five percent annual interest. First, put your variables into the present value equation. The completed equation is as follows: P V = $ 10 , 000 ( 1 + 0.05 ) 5 {\displaystyle PV={\frac {\$10,000}{(1+0.05)^{5}}}} PV={\frac {\$10,000}{(1+0.05)^{{5}}}} Solve the addition within parentheses first. This yields: P V = $ 10 , 000 ( 1.05 ) 5 {\displaystyle PV={\frac {\$10,000}{(1.05)^{5}}}} PV={\frac {\$10,000}{(1.05)^{{5}}}} Then, solve the exponent. This yields: P V = $ 10 , 000 1.276 {\displaystyle PV={\frac {\$10,000}{1.276}}} PV={\frac {\$10,000}{1.276}} Note that the result, 1.276 is a rounded figure. You will get a different final result if you do not round this number. Divide the last two numbers. Your result is $7,836.99. So, getting $10,000 in five years is like getting $7,836.99 now.
Using Time Value of Money Calculations

Understand the implications of the time value of money. These calculations make it clear that time is literally money. The value of the money you have now is higher than the same amount of money in the future. This is why you should know how to calculate the time value of money. It allows you to determine which investments are better, based upon not just how much money they return to you, but when they return it.

Decide between payments using present value. Present value can be used to determine whether a present payment of a certain value will end up being worth more or less than a future payment of a different value. For example, imagine that you have won the lottery and are offered either $1 million now or $2.5 million in ten years. Your money manager advises you that you can safely earn ten percent interest per year if you invest the money. Which payment should you take? The present value of the $1 million is, obviously, $1 million. This is only two-fifths of the monetary value of the later payment, though. However, the $2.5 million payment is being made in ten years, during which time your $1 million could be earning ten percent interest (assuming you didn't spend it). If you apply the present value equation, you find that the present value of the $2.5 million is only about $964,000. So, you would want to take the $1 million now and invest it. It will be worth almost $2.6 million in ten years.
Calculate the net present value of an investment. Present value calculations can also be used to analyze the profitability of business projects by using the concept "net present value." Net present value refers to the present value of projected sales revenues or interest earnings from a project or investment minus the present value of money invested in the investment or project. In this way, it is used to see whether or not the project will be profitable. Alternately, it can be used to determine susceptibility to interest rate fluctuations.
















Comments
0 comment